
(viii) Quadratic by quadratic with no common factor is many one.

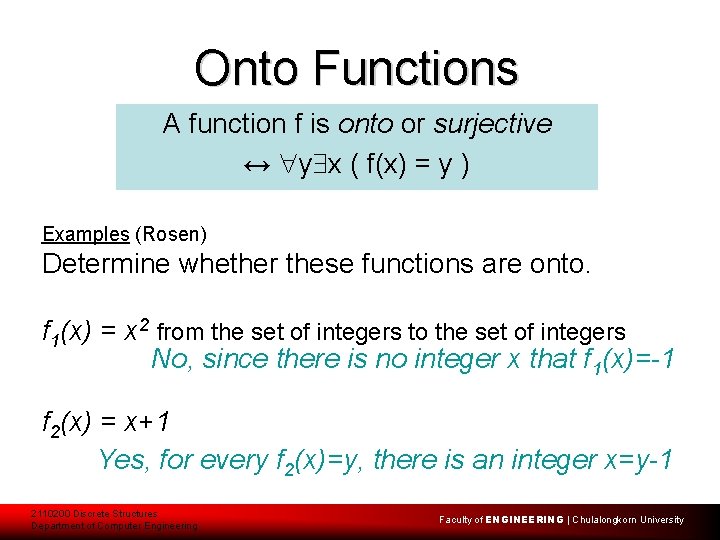
(vi) All even degree polynomials are many one. (v) All trigonometric functions in their domain are many one. (iii) If continous functions f(x) is always increasing or decreasing in whole domain, then f(x) is one-one. (ii) If any line parallel to x-axis cuts the graph of the functions atleast at two points, then f is many-one. (i) If a line parallel to x-axis cuts the graph of the functions atmost at one point, then the f is one-one. Hence its range is R \(\implies\) f is onto so f is bijective. Hence f'(x) always lies in the interval [1, \(\infty\)) Hence, f : Q \(\rightarrow\) Q is a bijection.Įxample : Let f : R \(\rightarrow\) R be a function defined as f(x) = \(2x^3 + 6x^2\) + 12x +3cosx – 4sinx then f is. That is every element in the co-domain has its pre-image in x. Onto (Surjective) : Let y be an arbitrary element of Q. Thus, f(x) = f(y) \(\implies\) x = y for all x, y \(\in\) Q. One-One (Injective) : Let x, y be two arbitrary elements in Q. Solution : We observe the following properties of f. Solution : Clearly, f is a bijection since it is both one-one (injective) and onto (surjective).Įxample : Prove that the function f : Q \(\rightarrow\) Q given by f(x) = 2x – 3 for all x \(\in\) Q is a bijection. for all y \(\in\) B, there exist x \(\in\) A such that f(x) = y.Īlso Read : Types of Functions in Maths – Domain and RangeĮxample : Let f : A \(\rightarrow\) B be a function represented by the following diagram : In other words, a function f : A \(\rightarrow\) B is a bijection, if it is Let’s begin – What is Bijection Function (One-One Onto Function) ?ĭefinition : A function f : A \(\rightarrow\) B is a bijection if it is one-one as well as onto.

Here, you will learn one one and onto function (bijection) with definition and examples.


 0 kommentar(er)
0 kommentar(er)
